Are your students ready to dive into the exciting world of volume? In this post, we will be discussing some effective strategies that you can teach your 5th grade students to find the volume of different objects. Since teaching volume can sometimes be a bit dry, I’m going to share some of my favorite strategies that are sure to keep your students motivated and eager to learn. So, let’s dive in and explore some of the best strategies for finding volume in 5th grade!
This post contains affiliate links. As an Amazon Associate I earn from qualifying purchases. These commissions help support the blog. All opinions are my own.
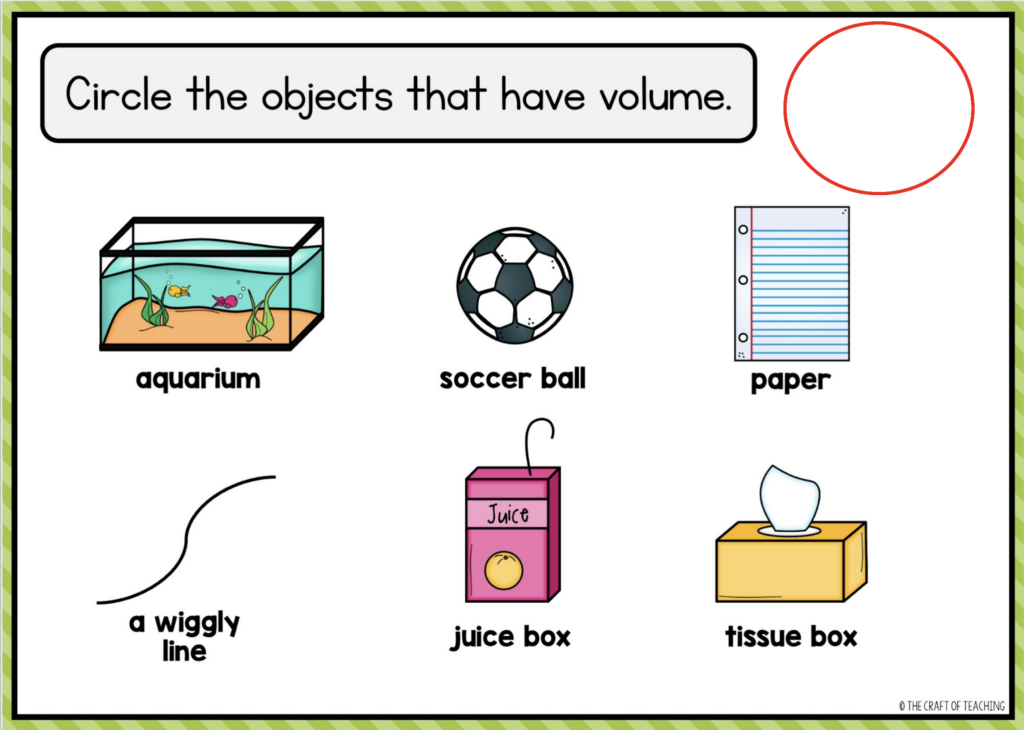
identifying objects that have volume
Before doing anything else, it’s important that students understand which types of objects have volume! Even though our unit in 5th grade focuses on right rectangular prisms, understanding that objects with volume take up space is essential. Most students can differentiate between 2D and 3D objects – but I’m always surprised when I ask them to identify objects with volume. It can be really tricky! We work on finding items in our classroom and determining if they have volume – the tissue box, the top of their desks, a piece of paper, etc. Sometimes, it’s hard when a 3D object is filled (like a box full of tissues), so I find these see-through geometric solids to be helpful!
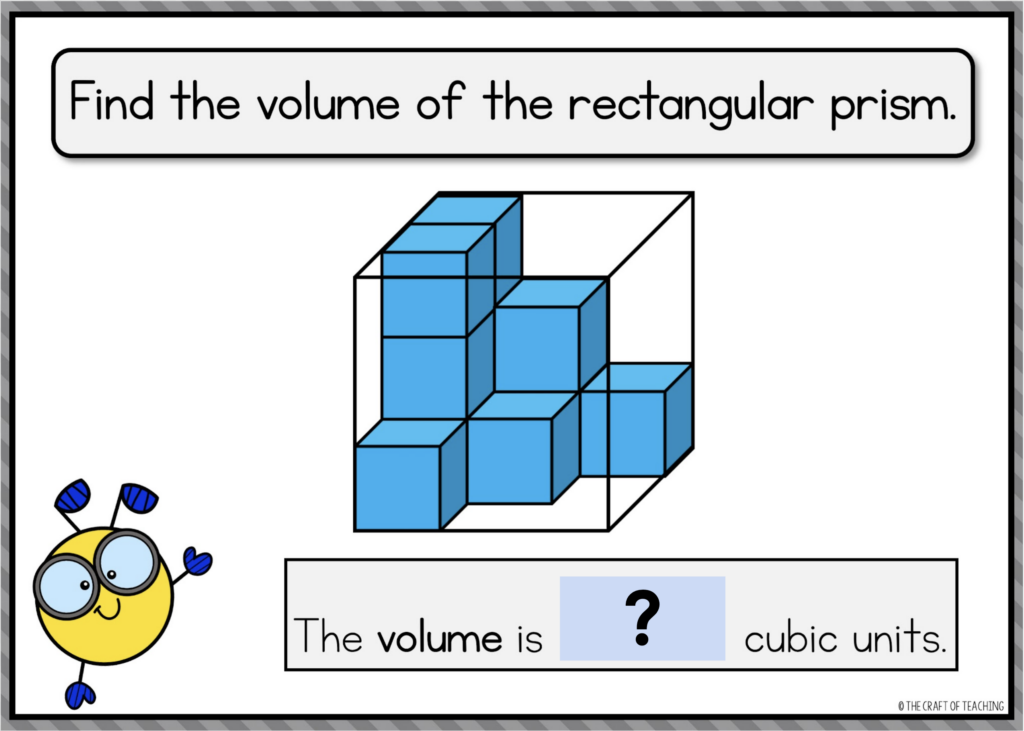
measuring volume by counting cubes
If we are going to talk about the best strategies for finding volume in 5th grade, we HAVE to talk about centimeter cubes! One of the first things to teach students when introducing volume is how to measure it by counting cubes. The best way I’ve found is to actually let them build right rectangular prisms with centimeter cubes and count how many cubes it takes to build ones of different sizes. Another fun way to practice finding volume by counting cubes is to build right rectangular prisms using nets, and pack the nets with cubes. This is a great way to reinforce the idea that the cubes have to fit exactly – no gaps and no overlaps – to be able to determine the volume.

Once students have had a chance to use the cubes in a hands-on way, they can begin to transfer their learning to representations on paper. Looking at images of prisms with cubes is a great way to stretch their thinking and help them visualize where all the cubes are in a prism when they can’t see them or count them directly. I always notice students coming up with more sophisticated strategies during this phase – looking at layers and counting, counting in groups, or even starting to make the connection to the formula for volume.

Measuring Volume By Iterating Layers
Most of the time, my students come up with this strategy (laying the foundation for the v=bXh formula) before I formally introduce it. They start to see the patterns in their earlier work and notice that they can count just one layer and multiply it by the number of layers in the prism. We usually talk about different perspectives when observing the prism: they can see the layers by cutting the prism vertically or horizontally, and look at it from the bottom, top, or sides. Students can use this strategy whether they are building prisms with cubes or if they are looking at drawings or representations of prisms.
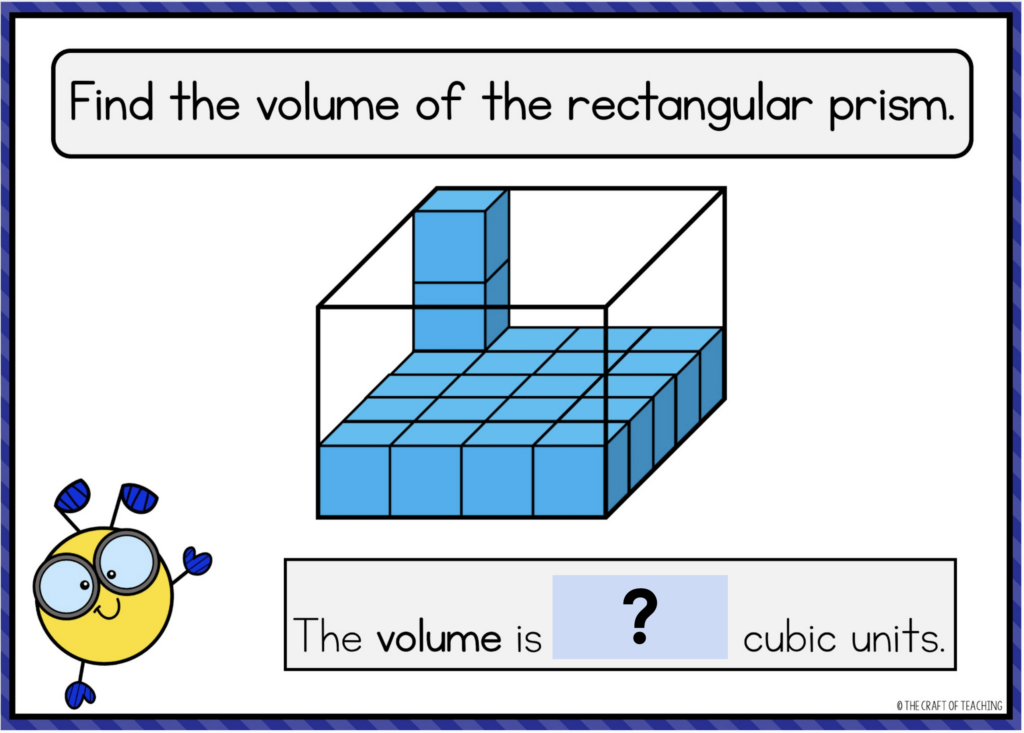
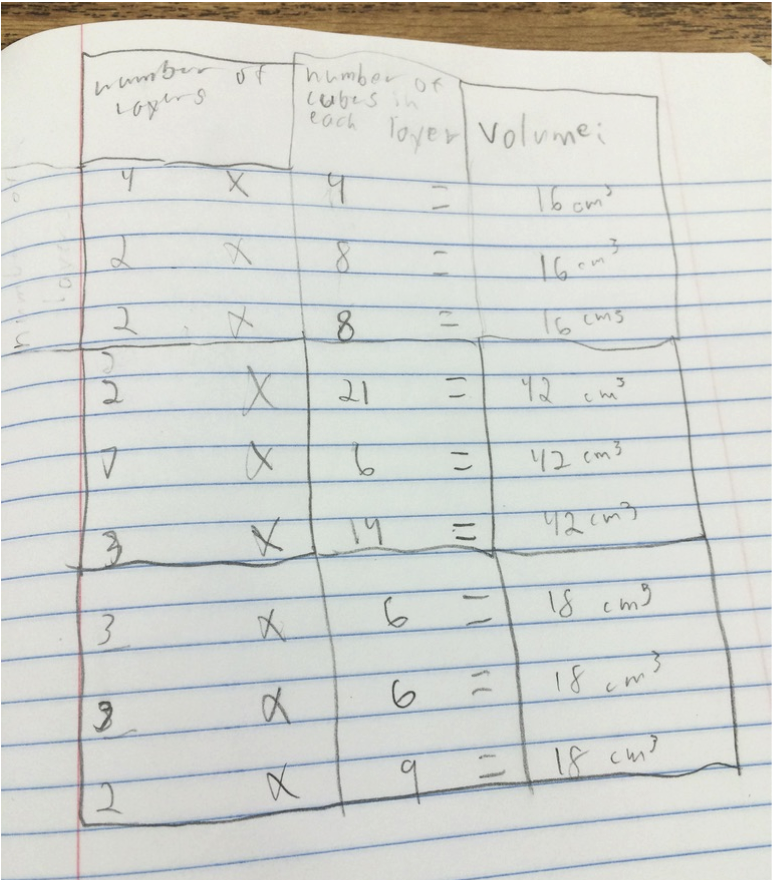
We make a chart with the number of layers, the number of cubes in each layer, and the total volume. Having the chart helps students keep track of how the multiplication results in the total volume and they can begin to see one way they can find volume easily. Once they notice that they can easily multiply using the layers, you can introduce the vocabulary words “base” and “height.”
introducing and using two volume formulas
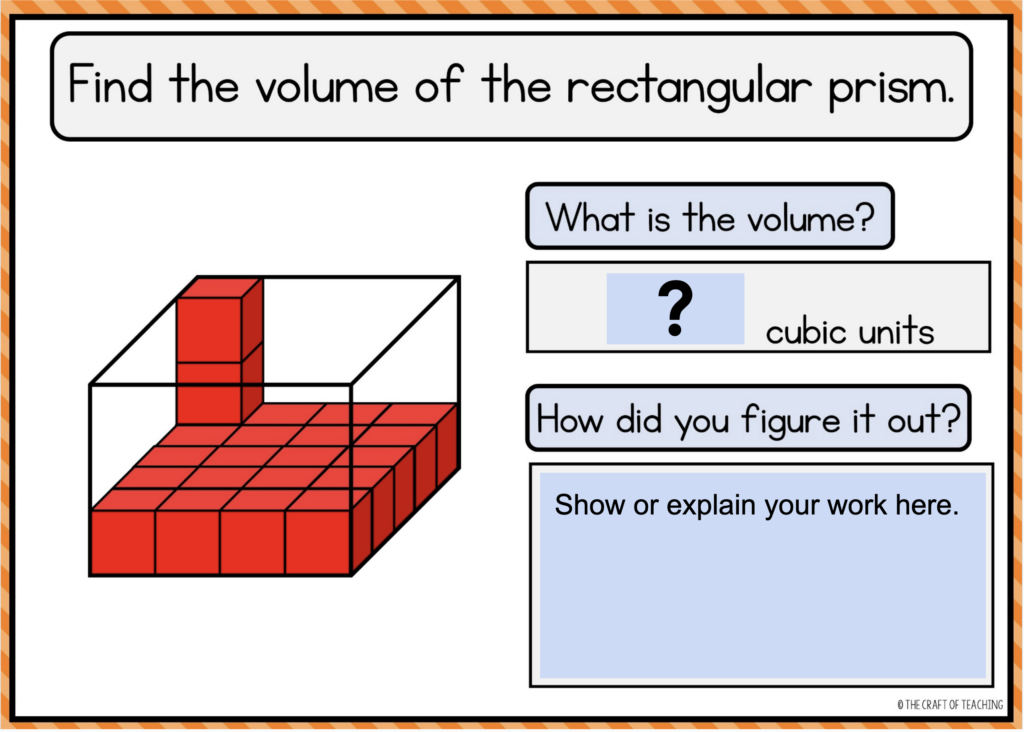
After students have built prisms, counted cubes, iterated layers and observed the length, width, and height of their prisms, it is time to make the jump to using volume formulas! Once your class has successfully used the layering strategy, it’s easy to introduce the first formula: base X height. Using the layers makes it easy for students to see how many cubes are in the base layer and how tall the prism is. (Even students who struggle with multiplication can use their addition skills to determine how many cubes are in all the layers put together.)
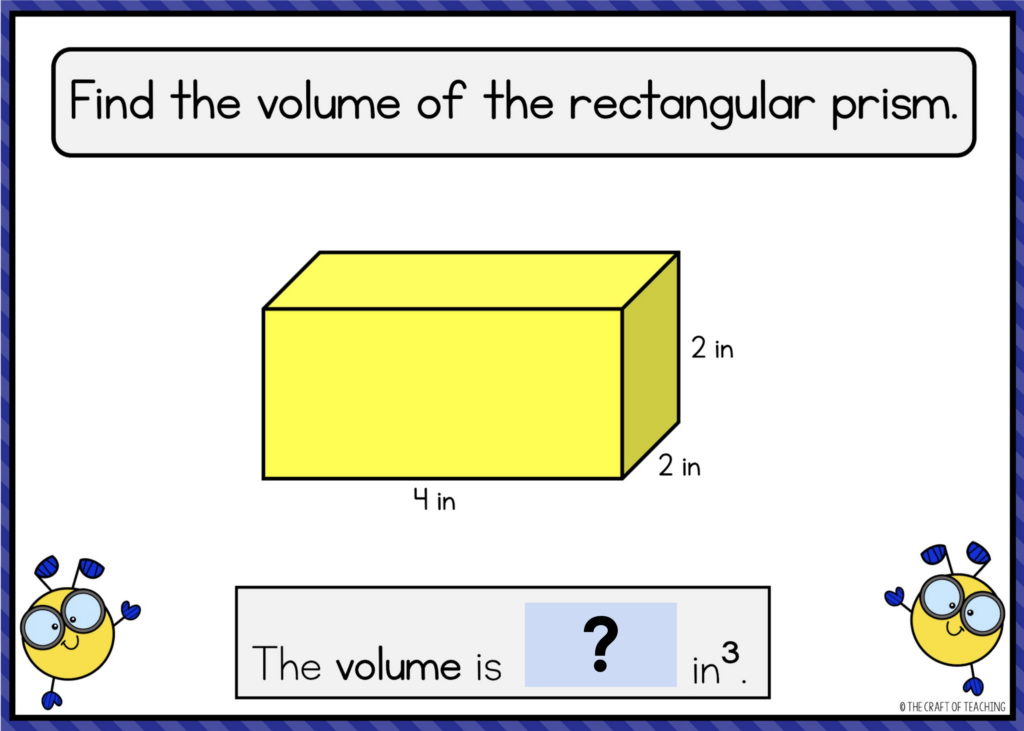
The traditional formula for volume (length X width X height) is sometimes trickier for students to discover. There are two ways to help student approach this strategy: use cubes to build prisms or connect to their prior learning about area.
If your students are more comfortable using the cubes, they can build prisms while recording their length, width, and height and total volume on a note catcher. From there, many students can make the connection that multiplying those dimensions results in the total volume!

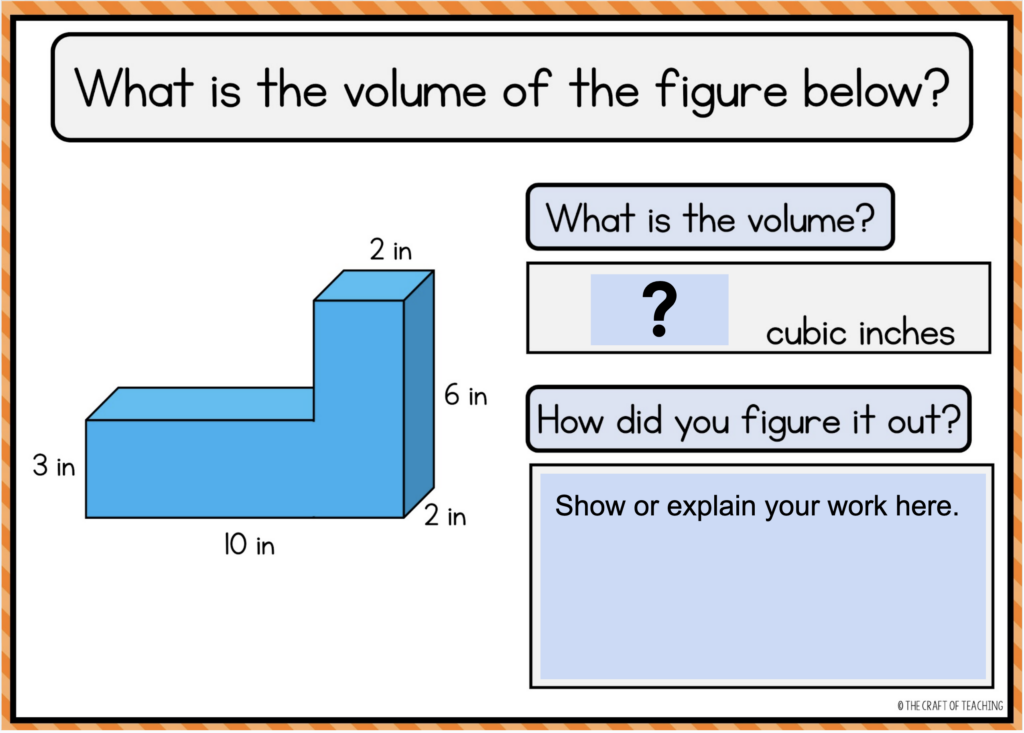
However, if your students are ready for a little bit more abstract thinking, you can have them analyze their observations from the base X height formula. If students notice that the base of the rectangular prism is a rectangle, they should already know that you can find the area of a rectangle by multiplying the length and the width. Once they know that the base = length X width, it’s easy to see that the missing dimension is height! From there, your class can use either formula to find the volume of rectangular prisms or even more challenging composite figures.
resources for practicing volume
Now that your students have the basics of finding volume down, they can have fun practicing! This digital volume resource is paperless, no prep, and perfect for your students to apply what they know about volume.
If you are ready to see what your students know about volume, Volume Quizzes in Google forms will be a useful tool. They are easy to assign in your learning management system and are automatically graded for you!


If you need both, you can pick up the bundle and save 20%!
You can also check out this blog post for more fun ideas (and two freebies!) about teaching and practicing volume with your students!
wrapping it up
And there you have some of the best strategies for finding volume in 5th grade! If you’ve got some tried and true strategies for this skill, I’d love to hear them in the comments below!
Don’t forget to head here and vote in the March Mathness bracket! You can download tons of freebies, vote for your favorite team, and enter to win an awesome giveaway!

Thanks for reading and voting! If you are interested in getting ideas and resources straight to your inbox, click here to start receiving my newsletter – I’ll send you some awesome math warm up ideas when you do!