But, inevitably, math is always the biggest loser. Maybe one or two hands shyly wave, but most kids avoid making eye contact at all costs.

One of the most concerning things I notice about 5th graders and math is the lack of confidence in their own abilities and thinking. At the beginning of the year it’s crucial to give students successful experiences to help them build their confidence and get ready to tackle harder, more complex mathematical situations.

Here’s one activity I use at the beginning of the year to help build mathematical confidence in my students.

This post contains affiliate links. I earn a small commission each time someone makes a purchase through one of these links. These commissions help support the blog. All opinions are my own.

Choosing A Problem

First, I choose a problem that I know every single kid (EVERY.SINGLE.ONE) can have access to. By this, I mean that the problem is open ended enough that even my most struggling students can participate and understand how to do something. (It doesn’t have to be grade level work here. Just a problem with enough entry points to let everyone dig in.)

One of my favorite resources for problems like these, is the book Eyes On Math: A Visual Approach to Teaching Math Concepts by Marian Small. This book is full of great visuals to help kids think about mathematical concepts. Each page has a visual aligned to a standard and a question to get kids thinking. The visual is also given as a pdf so you can print them or display them on your projector. There are teacher questions and ideas for extensions. Check it out {here.}

Working on the Problem
We started with a problem that is designed to explore factors.

At first, I give each student a copy of the picture. (Yes, I print them in color because my principal is nice, but you could print them in black and white and it would be just fine!) They have a few minutes to look it over by themselves. Then, I ask them what they notice about it and they record their “noticings” in their math notebook. They always notice the patterns and the colors – but they also notice that they are in three sections, there are 18 in each section and many kids notice that two of the sections have equal groups. I accept any and all ideas at this point, since I just asked them to “notice.”

Then I display the question that goes with the picture. In this case, it was “How many people can share 18 marbles?” They can use the picture to help them. I ask them to work alone for about 5 minutes and then they get together with a partner to share ideas. This also helps anyone who might be stuck to get on board and grab an idea. (In the years that I have done this, I have had very few kids who couldn’t engage on some level. If necessary, I get out beans so they can have a manipulative to work with.)

The best part about this section of the lesson is that many kids come up with one way to share – 2 people can each have 9 or 6 people can each have 3. But once they start talking to each other they quickly realize there are several answers! This is the best part in my opinion, because the conversations are amazing. Most kids think they are right (and they are) but to see that other people came up with different answers that are also right really gets them talking, thinking and proving their ideas.

After they talk to partners about their ideas we share out to the whole group and talk about all the different answers there could be. By this point, most of them have come up with all the possibilities, but just in case we discuss it and if there are any questions we use pictures of manipulatives to prove whether their ideas work.

Reflection
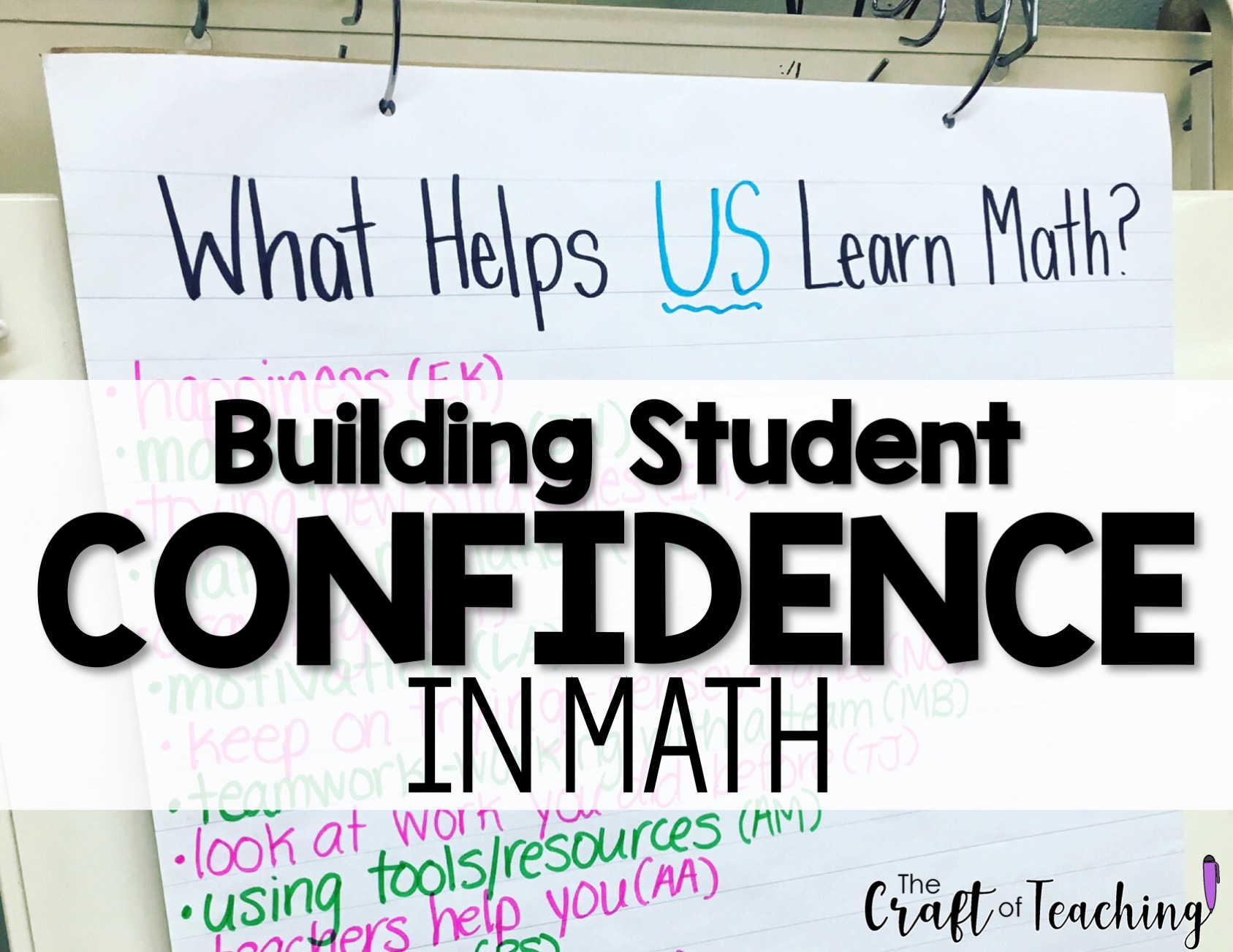
The last step in this process is reflecting. I ask each student to make a bullet list in their math notebook with the title “What Helps Me Learn Math.” I ask them to think back to the problem they just worked on and write down things that helped them be successful.

After everyone has made their lists, we use one idea from each person to create my absolute favorite anchor chart of the year. We do it together and it is a great way to honor all the different ways kids prefer to learn in math. I do this every year to show my students that I care about their learning as individuals, and that we can respect the fact that not everyone will learn the same way.

I leave this anchor chart up all year as a reminder.

Starting the year with an easily accessible problem and some reflection on how we all learn really helps build the climate for mathematical learning in my classroom. When students feel like they CAN do it, the confidence can be there right from the start! I love starting off with this before we jump into our prescribed mathematics resource.